How Interesting Is Volume and Surface Area
Volume surface and area; trust me they are very interesting. Further, in this article you will read about their qualities. Have you ever wrapped a birthday gift? If so, then you’ve covered the surface area of a polyhedron with wrapping paper.
Have you ever wrapped a birthday gift? If so, then you’ve covered the surface area of a polyhedron with wrapping paper.
Have you ever poured yourself a glass of milk? If so, then you’ve filled the volume of a glass with liquid.
Surface area is exactly what it sounds like — the area of all of the outside surfaces of a three-dimensional object. And volume is all of the space inside a three-dimensional object.
Read the below descriptions of volume surface and area!
INTRODUCTION:
Volume and surface area is the two basic units in Mathematics which are used to measure the region inside the 3-dimensional shapes. The 3-dimensional shapes are those which are having Length Width and Height.
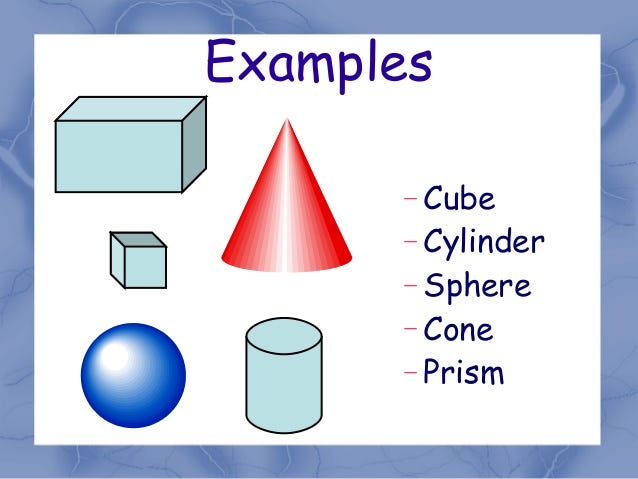
INTRODUCTION OF VOLUME:
Volume is basically used to calculate the amount of space inside a solid. Volume basically defines the space occupied by the mass.
The volume of an object is required three variables:
- Length of the object
- Width of the object
- Height of the object
The volume of the object in math basically deals with 3-dimensional shapes:
SOME PRACTICAL PROBLEM BASED ON VOLUME:
- CALCULATE THE VOLUME OF CUBE: A cube is 3-dimensional shapes. It is having Its length width and height. For Example:

Suppose Length = 5 meter
Width = 6 meter
Height = 7 meter
Volume of cube = Length x Width x Height
= (5 x 6 x 7) m3
= 210 m3
- VOLUME OF CYLINDER: Cylinder is also an example of 3 Dimensional shapes.

In the case of a volume of a cylinder sometimes we are only provided with Radius and height of the cylinder.Then volume of cylinder is calculated by this formula= πr2h
Π = 3.14 (The value of π (pi) never changed it is always 3.14)
r = Radius (Radius for every question is different)
h = Height (Height is also different for every case)

Here Radius = 5 meter
Height = 2 meter
Volume of cylinder = πr2h
= 3.14 x 52x2
= 3.14x25x2
= 157.08 m3
Here we wrote m3 because it is a three-dimensional shape and it is having three units.
VOLUME Formula of other 3 D Shapes:
- Volume of Pyramid =


- VOLUME OF CONE =
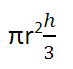

- VOLUME OF SPHERE =
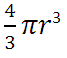

INTRODUCTION OF SURFACE AREA:
The surface area of a solid object is the measure of the total area that the surface of an object occupies. Surface area is the total area of the surface for a three-dimensional object. In simple words, we can say the surface area is the sum of all the areas of all the shapes covers the surface of the object.
The surface area of the object requires the things:
- Height of the object
- Depth of the object
Some Examples of Surface Area Shapes:

SOME PRACTICAL PROBLEM BASED ON SURFACE AREA:
- SURFACE AREA OF CUBE: A cube is three-dimensional symmetrical shapes and it is having 6 equal squares. Cube is also called Regular Hexahedron.

- Surface Area of cube= 6a2
Here “a” is the edge
Suppose “a” = 3 meter
= 6 (3)2
= 6×9
= 54 meter2
- SURFACE AREA OF CYLINDER:

The formula which we use to calculate the surface area of Cylinder is = 2πrh+2πr2
Where,
Π=3.14 (π (pi) value never changed for any question)
r = Radius
h = height
Suppose,
r = 2 cm
h = 4 cm
Now S.A. = (2×3.14x2x4) + (2×3.14×22)
= 75.4 cm2
- SURFACE AREA OF RIGHT RECTANGULAR PRISM:
S.A. of rectangular prism=2(bl+hl+hb)

Base=2m
Height=3m
Length=4m
S.A. = 2(2×4+3×4+3×2)
= 2(8+12+6)
= 2(26)
=52m2
- SURFACE AREA OF SPHERE:
S.A. =4πr2

- SURFACE AREA OF HEMISPHERE:
Hemisphere is the half of sphere so its surface area will be half of Surface area of Sphere.
S.A. of hemisphere= 2πr2

Sharing is Caring
