Inference on Linear Regression

Conditions for inference on slope (L-I-N-E-R)

It can be concluded as L-I-N-E-R:

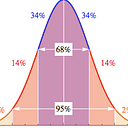
• L: Linear condition (Has linear relationship between x&y )
• I: Independent condition (Individual observations with replacement or 10% Rule)
• N: Normal condition (Sample size is at least 30)
• E: Equal variance condition
• R: Random condition

Confidence interval for slope

Here’s the formula for estimating the slope:

Notice:

• We’re using T-interval for estimating slope
• Degree of freedom(DF) becomes: n-2

Interpreting the output of Inference of Slope

Example

Solve:

• Interpret the table.
• Collect essential values for calculating CI:
• Expected value of slope
• T-value
• Sample size
• Calculate with formula

T statistic for Slope

Here is the formula for T statistic for slope:

Example

Solve:

Use CI to make conclusions about slope

▶︎ Back to previous note: Significance Testing

Normally, we can make conclusion simply by comparing P-value with Significance level.

But there’re cases ask us to make conclusion by comparing Confidence level with Significance level.
In that case, we can judge it by simply examine whether the Confidence intervalcovers 0 or not.

Since Confidence Level + Significance Level = 100%:

• CI exlcudes 0 ▶ Smaller interval & larger significance ▶ Significance level > P-value ▶ Not reject
• CI includes 0 ▶ Larger interval & smaller significance ▶ Significance level < P-value ▶ Reject

Example

Solve:

-